Abel's Proof: Step Two

In today's blog, I will cover step 2 of the original proof by Niels Abel on the quintic equation. The content in today's blog is taken from Peter Pesic's excellent book Abel's Proof.

In Step 2, Abel shows that if the general quintic equation has a solution expressible in radicals, then all irrational functions in this formula are expressible as rational functions of the roots.

This step was the gap in Paolo Ruffini's proof.

Lemma 1:

The equation:

[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁵ -a[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁴ + ... + e = 0

can be reduced to:

0 = q + q₁R^(1/m) + q₂(R^(2/m)) + ... + q_m-1R^(m-1)/m

where q, q₁, q₂, ... are rational functions based on the quantities a,b,c,d,e,p,p₂, ... and R.

Proof:

(1) We start with the following:

[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁵ -
a[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁴ + ... + e = 0

where a,b,c,d,e are rational coefficients.

(2) Since the equation does not involve any additional radicals, we can see that it can be ordered around sums of xR^(u/m) where x is a rational function of p,R,a,b,c,d,e and u is an integer.

(3) If u is greater m, then there exists q,r such that u=qm + r where r ≤ m-1.

(4) xR^(u/m) = xR^(qm+r)/m = xR^q*R^(r/m)

(5) If we set x' = x*R^q, then we have:

xR^(u/m)=x'R^(r/m) where r is less than m.

(6) So, if we number each of the x', then we are left with:

[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁵ - a[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁴ + ... + e =x'₀R^(0/m) + x'₁R^(1/m) + ... + x'_m-1R^(m-1)/m

where x_i is a rational function of a,b,c,d,e,p,p_i,R

QED

Corollary 1.1:

If R^1/m is not expressible in rationals, then q, q₁, q₂, ... all = 0.

Proof:

(1) Let z = R^(1/m)

(2) So, we have two equations:

z^m - R = 0

and

q + q₁z + ... + q_m-1z^m-1 = 0

(3) Using Abel's Lemma (see Lemma 2, here), we can conclude that z^m - R=0 is not reducible in rationals.

(4) Now, since R^(1/m) is a root for both equations, we can use Abel's Irreducibility Theorem (See Thereom 3 here) to conclude that q, q₁, ..., q_m-1 must all equal 0.

QED

Lemma 2:

If:





...



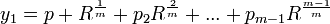
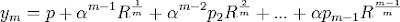
Then:

p = (1/m)(y₁ + y₂ + ... + y_m)

Proof:

(1) y₁ + y₂ + ... + y_m =

mp + (1 + α + α² + ... + α^m-1)R^(1/m) + p₂(1 + α + α² + ... + α^m-1)R^(2/m) + ... + p_m-1(1 + α + α² + ... + α^m-1)R^(m-1)/m

(2) Since (1 + α + α² + ... + α^m-1)=0 (see Lemma 2, here), we are left with:

mp = y₁ + y₂ + ... + y_m

(3) So that we have:

p = (1/m)(y₁ + y₂ + ... + y_m)

QED

Lemma 3:

If:





...



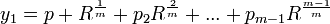
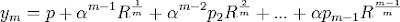
Then:

R^(1/m) = (1/m)(y₁ + α^m-1y₂ + ... + αy_m)

Proof:

(1) y₁ + α^m-1y₂ + α^m-2y₃ + ... + αy_m =

= (1 + α + α² + ... + α^m-1)p + mα^mR^(1/m) + p₂α^m(1 + α + α² + ... + α^m-1)R^(2/m) + .... + α^mp_m-1(1 + α + α² + ... + α^m-1)R^(m-1)/m

(2) Since α^m = 1 and (1 + α + α² + ... + α^m-1)=0 (see Lemma 2, here), we are left with:

mR^(1/m) = y₁ + α^m-1y₂ + α^m-2y₃ + ... + αy_m

QED

Lemma 4:

If:





...



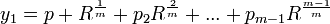
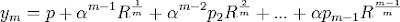
Then:

p_iR^(i/m) = (1/m)(y₁ + α^m-iy₂ + ... + αⁱy_m)

Proof:

(1) For any i, we have:

y₁ + α^m-iy₂ + ... + αⁱy_m =

= (1 + α + α² + ... + α^m-1)p + mα^m(1 + α + α² + ... + α^m-1)R^(1/m) + ... + p_iα^mR^(i/m) + .... + α^mp_m-1(1 + α + α² + ... + α^m-1)R^(m-1)/m

(2) Since α^m = 1 and (1 + α + α² + ... + α^m-1)=0 (see Lemma 2, here), we are left with:

mp_iR^(i/m) = y₁ + α^m-iy₂ + α^m-(i+1)y₃ + ... + αⁱy_m

QED

Theorem 5:

Let :



be a solution to the general quintic equation:

y⁵ - ay⁴ + by³ - cy² + dy - e =0

where p,p₂,..., p_m-1, R are expressible in radicals, m is a prime, and R^(1/m) is irrational.

Then the m roots are:





...



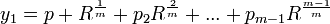
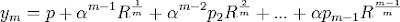
Proof:

(1) We can represent the general quintic equation as follows:

y⁵ + ay⁴ + by³ + cy² + dy - e = 0

(2) If we now insert this solution into the equation at step #1, we are left with:

[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁵ -
a[p + R^(1/m) + ... + p_m-1R^(m-1)/m]⁴ + ... + e = 0

(3) Using Lemma 1 above, we can reduce the above result to get:

0 = q₀ + q₁R^(1/m) + q₂R^(2/m) + ... + q_m-1R^(m-1)/m

where q₀, q₁, q₂, ... are rational functions based on the quantities a,b,c,d,e,p,p₂, ... and R.

(4) Using Corollary 1.1 above, we know that:

q₀, q₁, ..., q_m-1 all equal 0.

(5) Now, it is also clear that R^(1/m) has m different solutions where if R^(1/m) is one solution, the solutions are:

R, αR, α²R,..., α^m-1R where α is a m-th root of unity.

(6) So, if we use our equation for y, we are left with m roots:





...



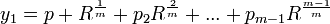
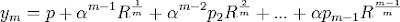
QED

Corollary 5.1:

Let :



be a solution to the general quintic equation:

y⁵ - ay⁴ + by³ - cy² + dy - e =0

where p,p₂,..., p_m-1, R are expressible in radicals, m is a prime, and R^(1/m) is irrational.

Then:

p,p₂, ..., p_m-1, R^(1/m) are rational functions of α, and the roots: y₁, y₂, ..., y₅

Proof:

(1) From Theorem 5 above, we have the m roots as:





...



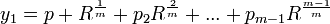
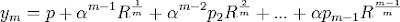
(2) Now, we complete this proof using Lemma 2, Lemma 3, and Lemma 4, since now we have:

p = (1/m)(y₁ + y₂ + ... + y_m)

R^(1/m) = (1/m)(y₁ + α^m-1y₂ + ... + αy_m)

p_iR^(i/m) = (1/m)(y₁ + α^m-iy₂ + ... + αⁱy_m)

QED

Corollary 5.2:

Let :



be a solution to the general quintic equation:

y⁵ - ay⁴ + by³ - cy² + dy - e =0

where each R is itself expressible in the same form such as:



Then:

there exists t, t_1,1, ... t_5,4 such that:

v^(1/n) = t + t_1,1y₁ + ... + t_1,4y₁⁴ + ... + t_5,1y₅ + ... + t_5,4y₅⁴

where v is any nested element of the above form.

Proof:

(1) If v is at the top level, then from Corollary 5.1 above, we know that:

v^(1/m) = (1/m)(y₁ + α^m-1y₂ + ... + αy_m)

(2) Likewise, if v is at the first nested level with R at the top level, then:



(3) Using the same logic as Corollary 5.1 above, we where treat R₁ = R, R₂ = αR, ..., R₅ = α⁴R, then we have:

v^(1/n) = (1/n)(R₁ + α^n-1R₂ + ... + αR_n)

(4) Then substituting the equation in step #1 above gives us:

v^(1/n) = (1/n)({(1/m)[y₁ + ... + αy_m]}⁵ + ... + α*α⁴{(1/m)[y₁ + ... + αy_m]}⁵)

(5) We can keep doing this substitution as far as needed so that we can assume that any nested form of v^(1/n) is a function of y₁, y₂, ..., y₅

(6) Finally, we can assume that no power is greater than m-1 since each root is a solution to the quintic equation and we can assume that:

y_i⁵ - ay_i⁴ + by_i³ - cy_i² + dy_i - e =0

(7) And further that:

y_i⁵ = ay_i⁴ - by_i³ + cy_i² - dy_i + e

QED

References

• Peter Pesic, Abel's Proof: An Essay on the Sources and Meaning of Mathematical Unsolvability, Appendix B, The MIT Press, 2004