In Step 2, Abel shows that if the general quintic equation has a solution expressible in radicals, then all irrational functions in this formula are expressible as rational functions of the roots.
This step was the gap in Paolo Ruffini's proof.
Lemma 1:
The equation:
[p + R(1/m) + ... + pm-1R(m-1)/m]5 -a[p + R(1/m) + ... + pm-1R(m-1)/m]4 + ... + e = 0
can be reduced to:
0 = q + q1R(1/m) + q2(R(2/m)) + ... + qm-1R(m-1)/m
where q, q1, q2, ... are rational functions based on the quantities a,b,c,d,e,p,p2, ... and R.
Proof:
(1) We start with the following:
[p + R(1/m) + ... + pm-1R(m-1)/m]5 -
a[p + R(1/m) + ... + pm-1R(m-1)/m]4 + ... + e = 0
where a,b,c,d,e are rational coefficients.
(2) Since the equation does not involve any additional radicals, we can see that it can be ordered around sums of xR(u/m) where x is a rational function of p,R,a,b,c,d,e and u is an integer.
(3) If u is greater m, then there exists q,r such that u=qm + r where r ≤ m-1.
(4) xR(u/m) = xR(qm+r)/m = xRq*R(r/m)
(5) If we set x' = x*Rq, then we have:
xR(u/m)=x'R(r/m) where r is less than m.
(6) So, if we number each of the x', then we are left with:
[p + R(1/m) + ... + pm-1R(m-1)/m]5 - a[p + R(1/m) + ... + pm-1R(m-1)/m]4 + ... + e =x'0R(0/m) + x'1R(1/m) + ... + x'm-1R(m-1)/m
where xi is a rational function of a,b,c,d,e,p,pi,R
QED
Corollary 1.1:
If R1/m is not expressible in rationals, then q, q1, q2, ... all = 0.
Proof:
(1) Let z = R(1/m)
(2) So, we have two equations:
zm - R = 0
and
q + q1z + ... + qm-1zm-1 = 0
(3) Using Abel's Lemma (see Lemma 2, here), we can conclude that zm - R=0 is not reducible in rationals.
(4) Now, since R(1/m) is a root for both equations, we can use Abel's Irreducibility Theorem (See Thereom 3 here) to conclude that q, q1, ..., qm-1 must all equal 0.
QED
Lemma 2:
If:
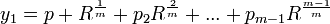

...
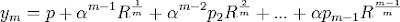
Then:
p = (1/m)(y1 + y2 + ... + ym)
Proof:
(1) y1 + y2 + ... + ym =
mp + (1 + α + α2 + ... + αm-1)R(1/m) + p2(1 + α + α2 + ... + αm-1)R(2/m) + ... + pm-1(1 + α + α2 + ... + αm-1)R(m-1)/m
(2) Since (1 + α + α2 + ... + αm-1)=0 (see Lemma 2, here), we are left with:
mp = y1 + y2 + ... + ym
(3) So that we have:
p = (1/m)(y1 + y2 + ... + ym)
QED
Lemma 3:
If:
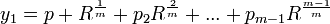

...
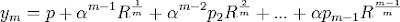
Then:
R(1/m) = (1/m)(y1 + αm-1y2 + ... + αym)
Proof:
(1) y1 + αm-1y2 + αm-2y3 + ... + αym =
= (1 + α + α2 + ... + αm-1)p + mαmR(1/m) + p2αm(1 + α + α2 + ... + αm-1)R(2/m) + .... + αmpm-1(1 + α + α2 + ... + αm-1)R(m-1)/m
(2) Since αm = 1 and (1 + α + α2 + ... + αm-1)=0 (see Lemma 2, here), we are left with:
mR(1/m) = y1 + αm-1y2 + αm-2y3 + ... + αym
QED
Lemma 4:
If:
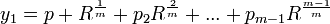

...
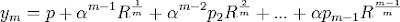
Then:
piR(i/m) = (1/m)(y1 + αm-iy2 + ... + αiym)
Proof:
(1) For any i, we have:
y1 + αm-iy2 + ... + αiym =
= (1 + α + α2 + ... + αm-1)p + mαm(1 + α + α2 + ... + αm-1)R(1/m) + ... + piαmR(i/m) + .... + αmpm-1(1 + α + α2 + ... + αm-1)R(m-1)/m
(2) Since αm = 1 and (1 + α + α2 + ... + αm-1)=0 (see Lemma 2, here), we are left with:
mpiR(i/m) = y1 + αm-iy2 + αm-(i+1)y3 + ... + αiym
QED
Theorem 5:
Let :

be a solution to the general quintic equation:
y5 - ay4 + by3 - cy2 + dy - e =0
where p,p2,..., pm-1, R are expressible in radicals, m is a prime, and R(1/m) is irrational.
Then the m roots are:
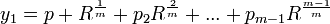

...
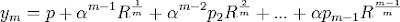
Proof:
(1) We can represent the general quintic equation as follows:
y5 + ay4 + by3 + cy2 + dy - e = 0
(2) If we now insert this solution into the equation at step #1, we are left with:
[p + R(1/m) + ... + pm-1R(m-1)/m]5 -
a[p + R(1/m) + ... + pm-1R(m-1)/m]4 + ... + e = 0
(3) Using Lemma 1 above, we can reduce the above result to get:
0 = q0 + q1R(1/m) + q2R(2/m) + ... + qm-1R(m-1)/m
where q0, q1, q2, ... are rational functions based on the quantities a,b,c,d,e,p,p2, ... and R.
(4) Using Corollary 1.1 above, we know that:
q0, q1, ..., qm-1 all equal 0.
(5) Now, it is also clear that R(1/m) has m different solutions where if R(1/m) is one solution, the solutions are:
R, αR, α2R,..., αm-1R where α is a m-th root of unity.
(6) So, if we use our equation for y, we are left with m roots:
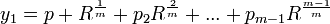

...
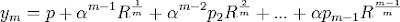
QED
Corollary 5.1:
Let :

be a solution to the general quintic equation:
y5 - ay4 + by3 - cy2 + dy - e =0
where p,p2,..., pm-1, R are expressible in radicals, m is a prime, and R(1/m) is irrational.
Then:
p,p2, ..., pm-1, R(1/m) are rational functions of α, and the roots: y1, y2, ..., y5
Proof:
(1) From Theorem 5 above, we have the m roots as:
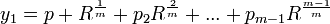

...
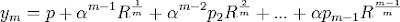
(2) Now, we complete this proof using Lemma 2, Lemma 3, and Lemma 4, since now we have:
p = (1/m)(y1 + y2 + ... + ym)
R(1/m) = (1/m)(y1 + αm-1y2 + ... + αym)
piR(i/m) = (1/m)(y1 + αm-iy2 + ... + αiym)
QED
Corollary 5.2:
Let :

be a solution to the general quintic equation:
y5 - ay4 + by3 - cy2 + dy - e =0
where each R is itself expressible in the same form such as:

Then:
there exists t, t1,1, ... t5,4 such that:
v(1/n) = t + t1,1y1 + ... + t1,4y14 + ... + t5,1y5 + ... + t5,4y54
where v is any nested element of the above form.
Proof:
(1) If v is at the top level, then from Corollary 5.1 above, we know that:
v(1/m) = (1/m)(y1 + αm-1y2 + ... + αym)
(2) Likewise, if v is at the first nested level with R at the top level, then:

(3) Using the same logic as Corollary 5.1 above, we where treat R1 = R, R2 = αR, ..., R5 = α4R, then we have:
v(1/n) = (1/n)(R1 + αn-1R2 + ... + αRn)
(4) Then substituting the equation in step #1 above gives us:
v(1/n) = (1/n)({(1/m)[y1 + ... + αym]}5 + ... + α*α4{(1/m)[y1 + ... + αym]}5)
(5) We can keep doing this substitution as far as needed so that we can assume that any nested form of v(1/n) is a function of y1, y2, ..., y5
(6) Finally, we can assume that no power is greater than m-1 since each root is a solution to the quintic equation and we can assume that:
yi5 - ayi4 + byi3 - cyi2 + dyi - e =0
(7) And further that:
yi5 = ayi4 - byi3 + cyi2 - dyi + e
QED
References
- Peter Pesic, Abel's Proof: An Essay on the Sources and Meaning of Mathematical Unsolvability, Appendix B, The MIT Press, 2004