Bombelli and the invention of complex numbers

Rafael Bombelli was the first to propose the idea of complex numbers.

Bombelli wrote about imaginary numbers in his very influential book Algebra which was published in 1572.

Bombelli based his argument for their usefulness on Girolamo Cardano's solution for the cubic equation. Consider the following equation:

x³ = 15x + 4.

This is an example of the depressed cubic so we can use del Ferro/Tartaglia solution (see here):

b=-15
c=4

with:



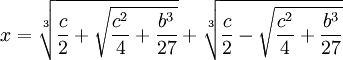
This then gives us:



So that:



which simplies to:



Cardano had considered such forms "irreducible" and had ignored them. Bombelli noticed that this wasn't quite right. For example, x³ = 15x + 4 is solved when x=4.

Bombelli proposed what he called a "wild thought". What if the term √-1 could be used mathematically so that the above value could be reduced to a number of the form: a+b√-1.

Bombelli proposed mathematical rules for allowing such numbers:

b₁√-1 + b₂√-1 = (b₁ + b₂)√-1

(b₁√-1)*(b₂√-1) = (-1)b₁b₂

and finally if:

a + bi = c + di, then a=c and b=d.

Then, using these assumptions, here's what he found:

(1) Assume that:

( a + b√-1)³ = 2 + √-121

(2) We note that (m + n)³ = m³ + n³ + 3mn(m + n) since:

(m + n)³ = m³ + 3m²n + 3mn² + n³ = m³ + n³ + 3mn(m + n)

(3) Let m = a, n=b√-1 which gives us:

( a + b√-1)³ = a³ + b³(-1)√-1 + 3(a)(b√-1)(a + b√-1) =

=a³ - b³√-1 + 3a²(b√-1) + 3ab²(-1) =

= a(a² -3b²) + b(3a² - b²)√-1.

(4) Now solving for ( a + b√-1)³ = 2 + √-121 gives us:

a(a² -3b²) = 2

b(3a² - b²) = 11

(5) Since 2,11 are primes, we know that in both cases, one of the two factors must be 1.

If we assume that a=2,b=1, then we see that:

2² - 3(1²) = 1
3*2² - 1² = 11

(6) So that:

(2 + √-1)³ = 2 + √-121.

(7) And using the same reasoning, we see that:

(2 - √-1)³ = 2 - √-121.

(8) If we assume that his reasoning is sound, we now find that:



reduces to:

x = (2 + √-1) + (2 - √-1) = 4

In this way, the theory of complex numbers was first presented by Bombelli and was eventually accepted by mathematicians.

References

• Paul J. Nahin, The Story of √-1, Princeton University Press, 1998