Abel's Impossibility Proof

Today's proof is taken from Peter Pesic's translation of Niels Abel's original 1824 proof. A special thanks to Peter for helping me by e-mail! :-)

Abel printed a limited set copies of his proof in hopes of using it as a "calling card" when he visited the great mathematicians of his day. Because he was short on funds, Abel was forced to keep the proof as short and concise as possible. He had hoped to secure recognition and financial sponsorship from his trip. When he ran out of money, he returned to his native Norway at the end of 1826.

In December of 1828, he grew very sick. He did not recover and died on April 6. Two days later, not knowing that he had died, a messenger arrived letting his family know that Abel had secured a mathematical position in Berlin. Abel was 26.

Abel was the second person to attempt this proof. His predecessor Paolo Ruffini came very close. So close, in fact, that Augustin-Louis Cauchy believed that he had succeeded. In his honor, the proof is often called the Abel-Ruffini Theorem. In fairness to Abel, Ruffini made an assumption in his proof which turns out to be true but which Ruffini did not prove.

In today's blog, I will show the argument in Abel's original proof of 1824.

Lemma 1:

Let:

(p + p₁S^(1/2))^(1/m) + (p - p₁S^(1/2))^(-1/m)

be a function on 5 parameters y₁, ..., y₅

where p,p₁,S are symmetric on the same 5 parameters and m ≥ 2.

Then:

this function returns m distinct values when its parameters are permuted

Proof:

(1) By Lemma 1, here, S^(1/2) takes 2 distinct return values when its parameters are permuted and we can call these two values s₁, s₂ and we know that s₁ = -s₂

(2) So, it follows that the two cases gives us two possible expressions:

(p + p₁s₁)^(1/m) + (p - p₁s₁)^(1/m)

and

(p + p₁s₂)^(1/m) + (p - p₁s₂)^(-1/m)


(3) But, clearly:

(p + p₁s₁)^(1/m) + (p - p₁s₁)^(1/m) =(p + [-p₁s₂])^(1/m) + (p - [-p₁s₂])^(1/m) =

= (p - p₁s₂)^(1/m) + (p + p₁s₂)^(1/m)

(4) So, it follows that swapping s₁ and s₂ does not change the value, so the number of return values when the m parameters are permuted is m.

QED

Theorem 2: A solution to the general quintic equation is not expressible by radicals.

Proof:

(1) Assume that a solution to the general quintic equation can be expressed in radicals. That is we can state an equation for each root y in terms of a,b,c,d,e such that:


y⁵ - ay⁴ + by³ - cy² + dy - e = 0


(2) If a solution to this equation exists, it can be represented as follows (see Theorem 5, here):



where m is a prime number and R, p₂, ..., p_m-1 are all functions of this same form finitely nested at the deepest level each p,p_i,R is a function of the coefficients of the general quintic equation.

(3) Using this form [see Theorem 5, here], we further note that the distinct roots of the quintic equation are expressible in terms of R:





...



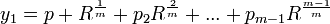
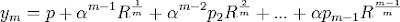
where α is an mth primitive root of unity [See here for a review of primitive roots of unity if needed]

(4) The Fundamental Theorem of Algebra gives us that the quintic equation has 5 roots. [See here for proof of the Fundamental Theorem of Algebra]

(5) Since this is the general solution, we can assume that each of the roots are distinct and that therefore, at the top level, m = 5.

(6) We can also state R^(1/5) in terms of the roots (this was the gap that Ruffini assumed but did not prove). Using Corollary 5.1 here, we note that:

R^(1/5) = (1/5)(y₁ + α⁴y₂ + ... + αy₅)

(7) Now, by our result in step #2, we can assume that this same equation holds true at all levels. So at the lowest levels, we can assume that R is a rational function of the coefficients a,b,c,d,e.

(8) We can also show that at the bottom level, R is a function of the 5 roots. [See Corollary 3.1, here]

(9) Then, from step #7 and step #8, using Corollary 6.1, here, we can conclude that at the depeest level, m = 2.

(10) Now, we have two states to consider. Either the expression in step #2 consists of only two levels (at the top level m=5 and at the bottom level m=2) or its consists of more than two levels.

(11) So, if there exists a level above the lowest level but below the top level, then by step #2 above, it must be characterized by a prime m and by step #8 above we can assume that it is a function of the 5 roots.

(12) From Lagrange's Theorem (See Theorem, here), we can assume that if we permuted the 5 roots, the number of return values will divide 5! = 120.

(13) From Corollary 1.1, here and step #9 above, we know that the number of return values will be 2*m. Since 2*m must divide 120 and m must be a prime, we conclude that m=2, 3, or 5.

(14) But m ≠ 5, since from the reasoning of Corollary 1.1 in step #13, if m=5, then ultimately we would have 2*5*5*n return values which does not divide 120.

(15) We also know that m ≠ 2 since this gives us 2*2=4 return values and by Cauchy's Theorem (see Theorem 2, here), if the return values are less than 5, then it is 1 or 2.

(16) Further, we know that m ≠ 3 since:

(a) Let r = (p + p₁S^(1/2))^(1/m)

where p,p₁,S are symmetric with respect to the 5 roots of a quintic equation.

(b) Let r₁ = (p - p₁S^(1/2))^(1/m)

(c) So, we define a function on the 5 roots of the quintic equation such that:

f(y₁, ..., y₅) = r + r₁

(d) From Lemma 1 above, such a function will return m distinct values when its m parameters are permuted.

(e) But, by Cauchy's Thereom used in step #15 above, if m is less than 5, it can only be 1 or 2.

(k) Hence, this means m ≠ 3.

(17) But, then it follows from steps #13, #14, #15, #16 above that the solution to the general quintic equation has only two levels and therefore the solution has the following form:



where m=5 and p,R,p₂, ... p₄ are functions of the form s + s₁T^(1/2) where s, T, s₁ are rational functions of the coefficients of the quintic equation: a,b,c,d,e.

(18) Now, from step #6 above, we have:

R^(1/5) = (1/5)(y₁ + α⁴y₂ + ... + αy₅)

where α is a primitive 5th root of unity and y₁, ..., y₅ are the 5 distinct roots of a quintic equation.

(19) Now, from #17 above, we have:

R^(1/5) = (s + s₁T^(1/2))^(1/5)

where s, s₁, T are symmetric on y₁, ..., y₅

(20) So, putting these two steps together gives us:

(1/5)(y₁ + α⁴y₂ + ... + αy₅) = (s + s₁T^(1/2))^(1/5)

(21) But this is clearly impossible since (1/5)(y₁ + α⁴y₂ + ... + αy₅) can take 5!=120 distinct return values when its the parameters are permuted and (s + s₁T^(1/2))^(1/5) can only take 2*5=10 distinct return values.

(22) Therefore, we have a contradiction and we reject our assumption in step #1.

QED

Corollary 2.1: A solution to the general n-th equation where n ≥ 6 is not expressible by radicals.

Proof

(1) Assume that a solution to a general n-th equation where n ≥ 6 is expressible by radicals.

(2) Let:

y⁵ - ay⁴ + by³ - cy² + dy - e = 0

be a general quintic equation.

(3) Let i = n - 5 so that i ≥ 1.

(4) If we multiply (y - c₁)*...*(y - c_i)*(y⁵ - ay⁴ + by³ - cy² + dy - e ), then we have an n-th equation.

(5) By assumption, we now solve this n-th equation so that our roots are y₁, ..., y₅, y₆, ..., y_n

(6) But then we have also solved the quintic equation using radicals which by Theorem 1 above is impossible.

(7) So, we have a contradiction and we reject our assumption in step #1.

QED

References

• Peter Pesic, Abel's Proof: An Essay on the Sources and Meaning of Mathematical Unsolvability, Appendix B, The MIT Press, 2004
• William Rowan Hamilton, "On the Argument of Abel", Transactions of the Royal Irish Academy, 18 (1839), pp 171-259.