Today's content is taken straight from Jean-Pierre Tignol's Galois' Theory of Algebraic Equations.
In the proofs below, I use σ(f) where f ∈ Q(μk)(μp) which is defined in Definition 6, here. For definition of Kf, see Definition 5, here.
Lemma 1:
Let ζ be a p-th period of unity.
Let ef=p-1
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Then:
σe(ηi) = ηi
Proof:
σe(ηi) = σe(ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i) =
=ζe+i + ζ2e+i + ζ3e+i + ... + ζef+i =
= ζe+i + ζ2e+i + ... + ζp-1+i =
= ζe+i + ζ2e+i + ... + ζe(f-1)+i + ζ0+i = ηi
QED
Corollary 1.1:
Let ζ be a p-th period of unity.
Let ef=p-1
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Let a ∈ Q
Then:
σe(aηi) = aηi
Proof:
σe(aηi) = σe(a[ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i ]) =
= σe(aζi + aζe+i + aζ2e+i + ... + aζe(f-1)+i ) =
= aζe+i + aζ2e+i + aζ3e+i + ... + aζef+i =
= aζe+i + aζ2e+i + aζ3e+i + ... + aζp-1+i =
= aζe+i + aζ2e+i + ... + aζe(f-1)+i + aζ0+i = aηi
QED
Lemma 2:
Let ζ be a p-th period of unity.
Let ef=p-1
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Then if ai ∈ Q:
σe(a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1) = a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1
Proof:
(1) σe(a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1) = σe(a0η0) + σe(a1η1) + σe(a2η2) + ... + σe(ae-1ηe-1) [From Definition 5, here]
(2) From Corollary 1.1 above, we have:
σe(a0η0) + σe(a1η1) + σe(a2η2) + ... + σe(ae-1ηe-1) = a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1
QED
Corollary 2.1:
if f(ζ), g(ζ) ∈ Kf, then: f(ζ)*g(ζ) ∈ Kf
Proof:
(1) Using Theorem 2, here, there exists a0, ..., ae-1 and b0, ..., be-1 such that:
f(ζ) = a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1
g(ζ) = b0η0 + b1η1 + b2η2 + ... + be-1ηe-1
(2) σe(f(ζ)g(ζ)) = σe(f(ζ))σe(g(ζ)) [See Lemma 6, here]
(3) σe(f(ζ))σe(g(ζ)) = σe(a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1)σe(b0η0 + b1η1 + b2η2 + ... + be-1ηe-1) = (a0η0 + a1η1 + a2η2 + ... + ae-1ηe-1)(b0η0 + b1η1 + b2η2 + ... + be-1ηe-1) =
= f(ζ)g(ζ) [This follows directly from Lemma 2 above]
QED
Lemma 3
Let ζ be a p-th period of unity.
Let ef=gh=p-1 where f divides g and k=g/f
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Let ω be a k-th root of unity
Then:
(η0 + ωηh + ... + ωk-1ηh(k-1))k = a0η0 + ... + ae-1ηe-1
where the coefficients a0, ..., ae-1 are rational expressions in ω over Q [that is, they are rational expressions in Q(μk)]
Proof:
(1) I will show that (η0 + ωηh + ... + ωk-1ηh(k-1))k can be expressed as
a0η0 + ... + ae-1ηe-1 where the coefficients a0, ..., ae-1 are rational expressions in ω over Q
(2) Assume that k = 1, then it is clear that a0 = 1, a1 = ω, a2 = ω2, ..., ak-1 = ωk-1
(3) Assume that our hypothesis in step #2 is true up to n such that k ≤ n, then the hypothesis holds for (η0 + ωηh + ... + ωk-1ηh(k-1))k
(4) Then (η0 + ωηh + ... + ωk-1ηh(k-1))n+1= (η0 + ωηh + ... + ωk-1ηh(k-1))n(η0 + ωηh + ... + ωk-1ηh(k-1))= (a0η0 + ... + ae-1ηe-1)(η0 + ωηh + ω2η2h + ... + ωk-1ηh(k-1))
(5) (a0η0 + ... + ae-1ηe-1)(η0 + ωηh + ω2η2h + ... + ωk-1ηh(k-1)) = (a0η0)(η0 + ωηh + ω2η2h + ... + ωk-1ηh(k-1)) + ... + (ae-1ηe-1)(η0 + ωηh + ω2η2h + ... + ωk-1ηh(k-1)) = a0η0ω0η0 + ... + ae-1ηe-1ωk-1ηh(k-1)
(6) It is clear that each of the resulting terms is in the form of aiηiωjηj*h so if I can prove that each of these form reduces to a rational expression of ω and ηi that I am done.
(7) aiηiωjηj*h = (aiωj)(ηiηj*h)
(8) Using Corollary 2.1 above and Theorem 2 here, this gives us that each term reduces to:
(aiωj)(b0η0 + ... + be-1ηe-1) where bi ∈ Q.
QED
Corollary 3.1:
Let ζ be a p-th period of unity.
Let ef=gh=p-1 where f divides g and k=g/f
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Let ω be a k-th root of unity
Then:
(η0 + ωηh + ... + ωk-1ηh(k-1))k = = a0η0 + ... + ah-1ηh-1 + ahηh + ... + a2h-1η2h-1 + + ... + + ah(k-1)ηh(k-1) + ... + ae-1ηe-1
Proof:
(1) From Lemma 3 above, we have:
(η0 + ωηh + ... + ωk-1ηh(k-1))k = a0η0 + ... + ae-1ηe-1
where the coefficients a0, ..., ae-1 are rational expressions in ω over Q [that is, they are rational expressions in Q(μk)]
(2) Since f divides g and ef = gh, it follows that g/f = e/h so that h divides e.
(3) This allows us to divide 0 .. e-1 into:
(η0 + ωηh + ... + ωk-1ηh(k-1))k = = a0η0 + ... + ah-1ηh-1 + ahηh + ... + a2h-1η2h-1 + + ... + + ah(k-1)ηh(k-1) + ... + ae-1ηe-1
QED
Lemma 4:
Let ζ be a p-th period of unity.
Let ef=gh=p-1 where f divides g and k=g/f
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Let ξj = ζj + ζh+j + ζ2h+j + ... + ζh(g-1)+j
Then:
ηi + ηh+i + ... + ηh(k-1)+i = ξi for i = 0, ..., h-1
Proof:
(1) ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i [From the definition above]
(2) ηi + ηh+i + ... + ηh(k-1)+i =
= [ ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i] + [ ζh+i + ζh+e+i + ζh+2e+i + ... + ζh+e(f-1)+i] + ...
+ [ ζh(k-1)+i + ζh(k-1)+e+i + ζh(k-1)+2e+i + ... + ζh(k-1)+e(f-1)+i] =
= [ ζi + ζh + i + ... + ζh(k-1)+i ] + [ ζe + i + ζh + e + i + ... + ζh(k-1)+e+i] + ...
+ [ζe(f-1)+i + ζh+e(f-1)+i + ... + ζh(k-1)+e(f-1)+i]
(3) Since k = g/f and ef=gh, it follows that e = gh/f = hk
(4) This then gives us:
[ ζi + ζh + i + ... + ζh(k-1)+i ] + [ ζe + i + ζh + e + i + ... + ζh(k-1)+e+i] + ...
+ [ζe(f-1)+i + ζh+e(f-1)+i + ... + ζh(k-1)+e(f-1)+i]=
[ ζi + ζh + i + ... + ζh(k-1)+i ] + [ ζhk + i + ζh + hk + i + ... + ζh(k-1)+hk+i] + ...
+ [ζhk(f-1)+i + ζh+hk(f-1)+i + ... + ζh(k-1)+hk(f-1)+i] =
[ ζi + ζh+i + ... + ζh(k-1) ] + [ ζhk+i + ζh(k+1)+i + ... + ζh(2k-1)+i] + ... + [ζh[kf-k] + i + ζh[kf-k+1] + i + ... + ζh[kf-1]+i]
(5) Since k = g/f, it follows that kf = g so that we have:
[ ζi + ζh+i + ... + ζh(k-1) ] + [ ζhk+i + ζh(k+1)+i + ... + ζh(2k-1)+i] + ... + [ζh[kf-k] + i + ζh[kf-k+1] + i + ... + ζh[kf-1]+i] =
[ ζi + ζh+i + ... + ζh(k-1) ] + [ ζhk+i + ζh(k+1)+i + ... + ζh(2k-1)+i] + ... + [ζh[g-k] + i + ζh[g-k+1] + i + ... + ζh[g-1]+i] = ξi
QED
Theorem 5:
Let ζ be a p-th period of unity.
Let ef=gh=p-1 where f divides g and k=g/f
Let ηi = ζi + ζe+i + ζ2e+i + ... + ζe(f-1)+i
Let ξj = ζj + ζh+j + ζ2h+j + ... + ζh(g-1)+j
Let ω be a k-th root of unity
Let t(ω) = η0 + ωηh + ω2η2h + ... + ωk-1ηh(k-1)
Then:
t(ω)k has a rational expression in terms of a0ξ0 + ... + ah-1ξh-1 where the coefficients a0, ..., ah-1 are rational expressions in ω over Q
Proof:
(1) By Corollary 3.1 above, we have:
t(ω)k = (η0 + ωηh + ω2η2h + ... + ωk-1ηh(k-1))k = = a0η0 + ... + ah-1ηh-1 + ahηh + ... + a2h-1η2h-1 + + ... + + ah(k-1)ηh(k-1) + ... + ae-1ηe-1
where the coefficients a0, ..., ae-1 are rational expressions in ω over Q
(2) Apply σh to both sides of the equation (see Lemma 7, here) gives us:
(ηh + ωη2h + ... + ωk-1ηh(k))k = (ηh + ωη2h + ... + ωk-1η0)k = = a0ηh + ... + ah-1η2h-1 + + ahη2h + ... + a2h-1η3h-1 + + ... + + ah(k-1)η0 + ... + ae-1ηh-1
(5) We note that ω-1(t(ω)) = ηh + ωη2h + ... + ωk-1.
(6) We further note that:
[ω-1t(ω)]k = (ω-1)kt(ω)k = (ωk)-1t(ω)k = t(ω)k
(7) So that it is clear that t(ω)k = (ηh + ωη2h + ... + ωk-1η0)k = = a0ηh + ... + ah-1η2h-1 + + ahη2h + ... + a2h-1η3h-1 + + ... + + ah(k-1)η0 + ... + ae-1ηh-1
(8) Since we can make the same argument for ω2t(ω) with σ2h applied, ω3t(ω) with σ3h, ..., ωk-1t(ω) with σh(k-1), we can make the following observation:
kt(ω)k = (a0 + ... + ah(k-1))(η0 + ... + ηh(k-1)) + + (a1 + ... + ah(k-1)+1)(η1 + ... + ηh(k-1)+1) + + ... + + (ah-1 + ... + ae-1)(ηh-1 + ... + ηe-1).
(9) Using Lemma 4 above that ηi + ηh+i + ... + ηh(k-1)+i = ξi for i = 0, ..., h-1, it follows that t(ω)k is rationally expressed in terms of ω and ξ0, ..., ξh-1 as:
t(ω)k = 1/k((a0 + ... + ah(k-1))ξ0 + ... + (ah-1 + ... + ae-1)ξh-1)
QED
Corollary 5.1: For every integer n, the n-th roots of unity have expressions by radicals
Proof:
(1) This is clearly true if n=1 or n=2 since the roots of unity are (1) or (1,-1)
(2) We may assume that for every integer k less than n, the k-th roots of unity are expressible by radicals
(3) If n is not prime, then since each of its factors are less than n, we can conclude that n is expressible by radicals (see Theorem 2, here).
(4) So, we can assume that n is prime.
(5) We can then order the n-th roots of unity other than 1 with the aid of a primitive root of n [see Theorem 3, here]
(6) We can then consider the Lagrange resolvent (see Theorem, here):
t(ω) = ζ0 + ωζ1 + ... + ωn-2ζn-2
where ω is an (n-1)-st root of unity.
(7) By the induction hypothesis, ω can be expressed by radicals.
(8) By the Theorem 5 above (with k = g = n-1), this shows that t(ω)n-1 has a rational expression in terms of ω
(9) We can then use Lagrange's formula (see Theorem, here), to get the following formula:
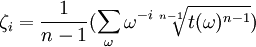
QED
References
- Jean-Pierre Tignol, Galois' Theory of Algebraic Equations
, World Scientific, 2001

No comments:
Post a Comment